
The North Carolina Gambit
March 2024
In this issue, you will find:
Martin Roper – Mistakes in Reinfeld
Charles Roberson – Connections Through Space and Time
John Timmel – To Move and Win!
Mistakes in Reinfeld – Martin Roper
I don’t think there is more “bang for the buck” when it comes to studying chess than practicing tactics. It develops pattern recognition and calculation, two of the most important skills in chess.
Two books that helped me the most as a young player were Fred Reinfeld’s 1001 Brilliant Ways to Checkmate and 1001 Winning Chess Sacrifices and Combinations. Fifty years ago these books propelled me from 1500 to over 1700.
Recently I went through them again and found a number of errors, omissions, or alternative or quicker solutions. I’ll go through these in a series of articles using BWTC and WCS&C and the problem number.
WCS&C #18, White to move:

WCS&C #54, White to move:

Answers:
WCS&C #18, Reinfeld gives, “1. Nd7!! Nxd7 2. Qxe6+ Qxe6 3. Bxe6+ Rf7 4. Bxf7+ and wins.”
But after 1…Nxd7 2. Qxe6+ the calm …Kh8 gives Black comfortable equality and maybe even more after 3. Qxf6 Nxf6. If White tries 3. Qxd7??, he’s won a piece but it doesn’t look like there is any way he can survive after …Rad8 4. Qb5 Re8+ 5. Be2 Qd4. White can try instead 2. Bxe6+ Kh8 3. Bxd7, but …Rf7 4. Bxf5 Re7 5. Bd3 Rxe2+ 6. Bxe2 Re8 looks lethal.
Probably best from the diagram is 1. Bd2 Re8 2. 0-0-0 with an edge for White but lots of chess ahead.
WCS&C #54, Reinfeld gives, “1. Qe3 Qb8 (1…Qd6 2. Nf6+ wins Black’s Queen; If 1…Bxd5 2. Rxd5 3. Qd4 wins the pinned Knight) 2. Re1 Bxd5 3. Bxd5+ Nf7 4. Rf1 and White wins the pinned Knight.”
The alternate solution I found was 1. Qf4 Nd7 2. Nc7 Qf8 3. Nxa8 Qxa8 4. Qd6 assessing that White is winning. If 4…Qe8 5. Re1 Nf8 6. Bd5 Kf7 7. Rf1+ Kg8 8. Rxf8+ with an easily-won endgame for White.
Connections Through Space and Time – by Charles Roberson
“I know a guy who knows a guy who knows a guy who knows a guy
who knows a guy who knows Kevin Bacon…” – Weird Al
There exists a mathematical theory that all 7 billion people in the world are connected by six degrees of separation or less. In other words, you can’t pick any two people on the earth that can’t be connected by 5 or less people. While this sounds like a small number, it may be correct. As an experiment, a package was dropped off at a random address in the other half of the world with instructions to mail this package to somebody you personally know that is closest to the specified final address. The path of the mailings was tracked and it arrived at the destination in 6 mailings not counting the original mailing to a random address. This doesn’t prove the hypothesis is correct, but it suggests that it is plausible.
While thinking about the 6 degrees of separation from anybody in the world, I thought that this applies through space but what about time? I have many connections with other scientists and chess players. I have Bachelor and Masters of Science degrees in Computer Science and have written chess programs that have entered several world computer chess championships (classical, Blitz, and Rapid time controls). So, I traced my connections to various people who died before I was born. One might think that the degrees of separation would be much larger when traveling through time. The number still seems small going back around 150 years.
Here is an example:
My Einstein number is my degree of separation from Albert Einstein which is 3: rather small due to my Shannon number of 2.
Charles Roberson -> Dr. Jaap Van Den Herik -> Dr. Claude Shannon -> Dr. Albert Einstein
Just count the arrows to get the number. In July 2002, I attended and my program Noonian Chess competed in the World Computer Chess Championships. While there I met many computer scientists doing research in the field including Dr. Jaap van den Herik, Dr. Tony Marsland, David Levy, and Dr. Johnathan Schaeffer. Almost every year, the World Computer Chess championships have an honored guest. When I was there it was Eugene Nalimov of Nalimov Endgame Tablebases fame. In some other events, Mikhail Botvinnik and Dr. Claude Shannon (famous American mathematician and scientist). Dr. Jaap van den Herik as well as David Levy, Dr. Tony Marsland and others met both Botvinnik and Shannon. Dr. Shannon has met many scientists including Albert Einstein and Dr. Alan Turing who is a famous mathematician that helped crack the enigma code during World War II. Also, a Hollywood movie was made about him: “The Imitation Game” starring Benedict Cumberbatch and Keira Knightley. Thus, my Turing number is 3.
Charles Roberson -> Dr. Jaap Van Den Herik -> Dr. Claude Shannon -> Dr. Alan Turing.
Some have made games of the idea like the 6 degrees of Kevin Bacon which postulates that there doesn’t exist a Hollywood actor that hasn’t worked with Kevin Bacon in a movie or hasn’t worked with him through 6 Degrees of Separation. The hypothesis seems to hold. For chess players, there is your Morphy number. Your Morphy number is how many people connect you to Paul Morphy. More on this here: https://en.wikipedia.org/wiki/Morphy_number
In most situations, your number to a person is through acquaintances. However, your Morphy number is linked to Paul Morphy via your chess games. The webpage lists several people with small Morphy numbers and shows Emanuel Lasker with a Morphy number of 2. So, my Morphy number via acquaintances is 5.
Charles Roberson -> Dr. Tony Marsland -> Mikhail Botvinnik -> Emmanuel Lasker -> somebody -> Paul Morphy
I say “somebody”, because the webpage says Lasker has a Morphy number of 2 without identifying the person. Now, for my Morphy number via played chess games:
Charles Roberson -> NM David Steele -> GM Bobby Fisher -> Botvinnik -> Lasker -> somebody -> Paul Morphy.
This gives a value of 6. According to the webpage, GM Hikaru Nakamura has a Morphy number of 4. He played NM Craig Jones who I’ve played. Thus, my Morphy number is still 6. Also, that gives me a Nakamura number of 2.
Other Examples via Acquaintances:
My Steinitz number is 4 (via acquaintances): Charles Roberson -> Dr. Tony Marsland -> Mikhail Botvinnik -> Emmanuel Lasker -> Wilhelm Steinitz
My Will Smith (Actor) number is 2: Charles Roberson -> GM Maurice Ashley -> Will Smith
My Stevie Nicks (Singer Fleetwood Mac) is 2: Charles Roberson -> Dr. Walter High -> Stevie Nicks
My Bill Gates (Microsoft co-founder) is 2: Charles Roberson -> Elwood Costlett -> Bill Gates.
You can see the world may be much smaller than most think. Thus, subcultures like the chess world could be very small.
New Party Games
From this, I’ve created two party games:
Famous People Group Number
In this game, your party attendees pick a list of famous people. Let’s say each attendee picks one famous person for the list. Then each attendee calculates their connection to each person in the list. Your score is the sum of those connections. The winner of the game is the person with the lowest sum. If you don’t know your connections to a person on the list then you could use a maximum value of 6 or be smart: use the lowest value in the room and add 1.
The game would start with totals for each participant then many totals could drop as people people announce their numbers. Let’s say the list is Albert Einstein, Alan Turing, Robert Oppenheimer, Richard Feynman, Will Smith, Stevie Nicks and Bruce Lee. As you can see from my connection graph below, my Einstein number is 3, my Turing number is 3, my Oppenheimer number is at most 4, my Feynman number is at most 4, my Will Smith number is 2, my Stevie Nicks Number is 2 and my Bruce Lee number is 2.
So for that list of people my group number is 20. Does anybody have a smaller group number for that list of people?
Chess Player Numbers
There are two numbers: the acquaintance number and the chess games number. Here is the game. Pick a random person from the NC Chess players list and everybody playing calculates their acquaintance and game numbers for that person.
- Pick a random number between 1 and 1000.
- Go to ncchess.org, hover over the resources tab on the left and click top rated players.
- Look at the player listed by the number you picked between 1 and 1000.
- Now find the minimal number of games you have played to connect with him/her.
The USCF has a webpage that can help you recall your opponents and how many times you’ve played them.
- Go to this page https://new.uschess.org/civicrm/player-search and look yourself up
- At your profile page, scroll down and click “Show Game Statistics”
- Click “All” and “Top 30 Opponents”, then click “Search”.
That will display 2 columns: the top 30 opponents you’ve ever played by a number of games for your chess career and the top 30 opponents you’ve played by a number of games in the last year. This will help you trace your game links to any other chess player. For more info than that, you’ll have to look at all of your tournament records kept by the USCF. For those of us that have games from the early 90s and before, those records are missing.
Incomplete Graph
This is an incomplete graph of my acquaintances. It only includes people who link me to various scientists, chess champions, actors and singers. In Mathematics and Computer Science this is called an undirected graph. Such graphs have nodes and edges. The nodes are circles and the edges are lines connecting the circles. In this graph, the nodes represent people and the edges represent direct connections between two people. If you count the number of edges between two people, you get their connection level. There is a catch: many people are connected via multiple pathways, so you must find the shortest path between them. Graph Example
To Move and Win! by John Timmel
Event “chess.com SpeedChess 2023”
Date “2023.09.22”
White “Carlsen, Magnus”
Black “Nakamura, Hikaru”
EventDate “2023.09.04”
1. e4 e5 2. Nf3 Nc6 3. Nc3 g6 4. d4 exd4 5. Nxd4 Bg7 6. Be3 Nge7 7. Qd2 d6 8.
O-O-O h6 9. Be2 Be6 10. Rhe1 Qd7 11. Nxc6 Nxc6 12. Nd5 a6 13. f4 O-O-O 14. Bf3
Kb8 15. Kb1 Rhe8 16. Bf2 Rc8 17. Bh4 Nd4 18. Nf6 Bxf6 19. Bxf6 Nxf3 20. gxf3
Bxa2+ 21. Kc1 Be6 22. h4 Qb5 23. Qd3 Qa5 24. Qc3 Qh5 25. b3 Ka8 26. Kb2 Qb5 27.
h5 Qxh5 28. Rh1 Qb5 29. Rxh6 a5 30. Rg1 b6 31. Rg5 c5 32. Rh2 a4 33. Rd2 axb3
34. cxb3 Kb7 35. Rxd6 Ra8 36. f5 Qf1 37. fxe6 Ra1? 38. Qc2 Rea8 39. Rd7+ Kc6
White to move and win**
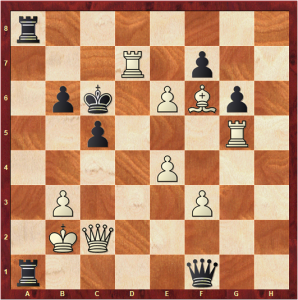
40. Rxc5+ bxc5
41. Rc7+ Kxc7
42. Qxc5+ Kb7
43. Qd5+ Kb8
44. Be5+ 1/2-1/2 ** with the draw, white clinched the tournament win.
The game could have concluded with
44. … Ka7 45. Bd4+ Kb8
46. Qd8+ Kb7 47. Qd7+ Ka6 48. Qc6+ Ka5 49. Qb6#